What does Perfect Play mean for Video Poker?
Simply put, perfect play means holding cards of a given hand that lead to the best possible expected value. Expected value is the average value of all possible outcomes. In the long-run, perfect holding gives the best average return. A perfect hold is also called an optimal hold. Our apps always show perfect holds.
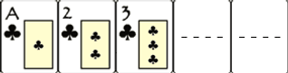
How important is this? Suppose you were dealt the following cards in a Jacks or Better video poker game:

What should you hold? The best hold depends on the game AND on the payouts for the various types of outcomes. For one common set of Jacks or Better payouts (often called the full-pay or 9-6 payouts) the best hold is:
Surprisingly, for another pay table (called the 9-5 pay table) where the only difference is that a Flush pays 5 coins per coin wagered instead of 6, the best hold is:
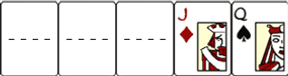
Had a player chosen this latter hold when playing the 9-6 game, the expected outcome would be 7.13% lower. That adds up! Furthermore, many players choose far worse non-optimal holds. Our apps hope to rectify that situation.
If you think this is an isolated case, you would be wrong. Fully 11,568 hands (627 not counting suit permutations of hands) should be played differently between these two pay tables.
What about Multiple Best Plays?
Surprisingly, it may be the case that multiple hold hands can lead to the same expected value of outcomes
Hold Hands with Equal Expected Values
Consider the video poker game Deuces Wild. With certain payouts (i.e., for the pay table labeled 800-200-25-15-9-4-4-3 having an Expected Value, i.e., EV, of 98.91%), the following hand has two different holds giving the same optimal expected value.

One discards the ten of Spades:

and the other discards the Jack of Hearts

Although these both give the same expected payout, the standard deviations - the potential spread of the outcomes - is much higher for the latter case. This is easy to see for this case. If the first hold is held, the worst possible outcome is Four of a Kind (paying 4 units) and the best, Five of a Kind (paying 15 units). If the second option is held, the worst outcome is Three of a Kind (paying 1 unit) and the best a Wild Royal Straight Flush (paying 25 units). The former case has a smaller low to high possible spread of payouts than the latter case.
The technical details are as follow. Both holds give an expected outcome of $4.9362 for every dollar wagered. So, over the long run, both holds are equivalent. However, the standard deviation for the first hand is $3.0694 and for the latter, $7.1798. Conservative players may prefer the lower spread case where others may want to "go for it" hoping for the higher outcomes - well, this is gambling after all. Of course, they may end up with a lower worse case. In the short run, only luck dictates who would be better-off.
Our professional apps will recommend the former hold when the default option "break expected value ties using the one with a smaller standard deviation" is set in Settings. Otherwise, the maximum standard deviation hold will be shown. (The player can toggle between the two and examine a table showing all the trade-offs)
The Focused Apps will accept either hold as acceptable.
Hold Hands with Equal Expected Values and Standard Deviations
In other cases there are equal expected values AND equal standard deviations, so one is really indifferent between which to play. In this case the Full Featured apps break ties in its recommendation by choosing the hand with the greater or fewer number of cards according to a user-selected setting. For example, in Jacks or Better, the following hand has two optimal hold hands:

One can hold the four Aces or one can hold all five cards. The Full Featured apps let you toggle between the two, but uses the recommended hand in the Game Play mode to track optimal play winnings.
Hold Hands with Equal Expected Values, Standard Deviation, and Number of Cards
Unbelievably, perhaps, there may still be equivalent tied, optimal hold hands. For certain Deuces Wild games, the following hand yields two optimal hold hands having the same expected value of outcomes, the same standard deviations and the same number of hold cards:

The two equivalent hold plays are hold the fours or hold the fives. The Full Featured apps break these ties using the user selected setting for either choosing the hand having the lower denomination cards (i.e., the pip) or the one with the higher one. The app lets you toggle between the two.
Very rarely - we know of only two cases across all our games and pay tables - there can be a three way tie. For example in Joker Poker (2 Pair) with pay table 100-800-100-100-16-8-5-4 (having an EV of 97.19%), for the hand:

The following WHY? partial listing shows the three tied hold hands.

The other hand is:

What does Perfect Play mean for Ultimate X Games?
Perfect Play for Ultimate X games is a bit harder to explain than is Perfect Play for regular Video Poker games. The reason is that what one does with the current hand not only affects what will be the immediate return, but also what the next round of play might yield since the outcomes of the current hands establish multipliers for the next round of hands.
Naïve Expected Value: The Naïve Expected value of a hand is the optimal expected outcome of all the hands times their current multipliers without regard for any future impact from the resulting new multipliers. This is Perfect Play ONLY for your last hand of play, since you are indifferent to any future impact. It is NOT Perfect Play in general. In fact, on your last hand of play you should only bet 5 units, not the max bet of 10 units since you are not interested in establishing new multipliers.
Perfect Play Expected Value: The expected value of Perfect Play takes future returns into account. The academic paper linked below gives the math behind a complete explanation (see Equation 1). In short, one must add a factor to the Naïve Expected return of a hold hand that captures both the probabilities of going to various different multiplier sets and the optimal expected returns of possible future hands.
Suppose you are playing 10 Line Jacks or Better with the following pay table and multipliers:

Now suppose you are dealt the following hand:

Suppose the current multipliers were all one, meaning the sum of the multipliers is 10. The WHY? button gives us the following information. We see that Perfect Play would have us hold the Ace, Seven and Eight of Spades. The Expected Value is 1.0471. This includes the current outcomes plus future potential outcomes. If this was our last hand of play, we see that the Naïve Expected value for holding just the Ace of Spades is higher than holding the three Spades (0.4642 versus 0.4468).

However, if the sum of current multipliers is 83, the WHY? button shows the following. Holding just the Ace of Spades is a Perfect Play (actually even if this is the last hand of play since its naïve expected value is also higher).

This example illustrates several things. Perfect Play is dependent not only on the dealt hand, but also on the sum of the current multipliers and on whether or not this is the last hand of play.
Multiple Best Perfect Play Holds
As for typical Video Poker games such as Jacks or Better, it may be the case that multiple hold hands can lead to the same expected value of outcomes. Indeed, in the example above when the multipliers summed to 83, the expected returns of the top two hold hands are very similar (33.0163 versus 33.0082), although not identical. Instead of repeating the arguments used for Video Poker games, we just refer the reader to the material above. However, breaking ties using the variance is problematic for Ultimate X because computing the variance not only depends on the exact multipliers (not just the sum of the multipliers) but is also very complex and time-consuming.
Thus, we break expected value ties using the variance or standard deviation of the naïve return. The standard deviation is just the square root of the variance and is often easier to work with because it has units in common with the expected value. The user can use the standard deviation of the naïve return as a judge of possible volatility of outcomes. Even this is complicated by the fact that the standard deviation of the naïve return depends on the actual multipliers, not on the sum of the multipliers. When we know the actual multipliers (for example, in the Game Mode), we can compute the exact standard deviation of the naïve return. Just take the square root of the sum of each multiplier squared and multiply the naïve standard deviation by this value.
When all we know is the sum of the multipliers, we compute a range of standard deviations. For example, in the 10 line Jacks or Better game above, suppose the sum of the current multipliers is 22. This might have come from multipliers (4,4,4,4,1,1,1,1,1,1) or from (4,2,2,2,2,2,2,2,2,2,2) or from (7,7,1,1,1,1,1,1,1,1), and possibly others. The base standard deviation for just one outcome would be multiplied by the square roots of 70, 52 and 106, respectively, for these three cases. Clearly, the standard deviation (the possible spread) of outcomes is greatest for (7,7,1,1,1,1,1,1,1,1), and least for (4,2,2,2,2,2,2,2,2,2,2). We show the range of these possible values in the Why? listing.
If the expected values and naïve standard deviations are tied, we break ties as in Video Poker games.
Math of Ultimate X and Bonus Streak Games
Below are links to academic analyses of Ultimate-X (mentioned above) and Bonus Streak Video Poker by our main scientist..
